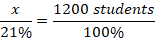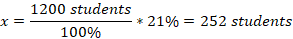A recipe calls for 2/9 cup of flour for every 1 2/5 cup of milk. To make a bigger batch, the chef uses 2 cups of flour. Which of the following would be the amount of milk needed for the bigger batch?
A. 12 1/5 cups
B. 12 3/5 cups
C. 8 1/25 cups
D. 10 3/25 cups
The number of cups of milk to make a bigger batch, we proceed as follows:
First convert the mixed number 1 2/5 as
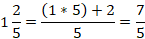
Then, we let the unknown number of cups of milk to be x and we set a proportion equation with number cups of floor as numerator and cups of milk as denominator
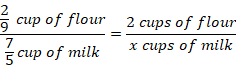
Find the value of x by cross-products
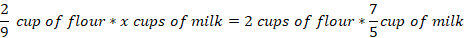
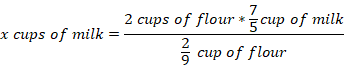
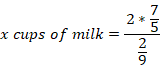
2 as a fraction is 2/1 which is then used to find the product of the numerator as follows.
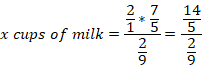
Per means division. The above equation becomes
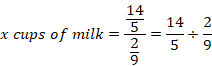
When dividing fractions, we multiply the first fraction with the reciprocal of the second. The reciprocal of 2/9 is 9/2. Thus
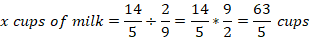
The numbers of cups of milk required is 63/5, which when converted to mixed fraction becomes 12 3/5.
Therefore, the Correct Answer is B.
More Questions on TEAS 7 Math
-
Q #1: Which of the following is the median of the date set below? 3, -5, 9, -1, 0
A. 9
B. -5
C. -1
D. 0
Answer Explanation
The median of a data set is a number that falls in the middle of the data set. To find the median, the numbers in the data set are arranged from the smallest to the largest. In the given data set, the organized arrangement is:
-5, -1, 0, 3, 9
There are five elements in the data set, and the median falls in the third position from either side. Thus, 0 falls in the third position.
-
Q #2: A person weighed themselves at 153 lb. Five months later they weighed themselves at 120 lb. Which of the following is the percent of weight the person lost over 5 months? (Round to the nearest percent.)
A. 38%
B. 22%
C. 19%
D. 29%
Answer Explanation
The percentage change in weight is found in three steps below:
Absolute change in weight=final weight-initial weight
Absolute change in weight= (left|120-153 ight|=left|-33 ight|=33)
Relative change in weight= (frac{absolute change}{initial weight}=frac{33}{153}=0.216)
Percent change=relative change * 100%
Percent change=0.216*100%=21.6%
The percent change in weight lost is 21.6 %, which is about 22%.
-
Q #3: There are 1200 students enrolled in four allied health programs at a local community college. The percent students in each program are displayed in the pie chart. Which of the following is the number of students enrolled in the radiologic technology program?
A. 216
B. 504
C. 228
D. 252
Answer Explanation
We are asked to find the number of students enrolled in the radiologic program using the information provided on the pie chart.
If we let x represent the number of students enrolled in the radiologic program, we set a proportion equation with number of students on the numerator and percentages on the denominator.
Total percent in the pie chart adds to 100%, which equals 1200 students. Then, 21% will represent
We solve the value of x by cross-multiplying the equation above.
Therefore, 252 students will enroll for a radiologic program.
